The paraxial wave Helmholtz equation describing the propagation of classical electromagnetic waves close to the optical axis is formally equivalent to the Schrödinger equation of quantum mechanics in two spatial dimensions. This concept has long been exploited to emulate certain aspects of quantum physics using light confined in waveguides, where the refractive index contrast plays the role of the potential in the Schrödinger equation. This has become even more interesting as a complex refractive index material allow to emulate non-Hermitian evolutions.

Our aim is to elevate this correspondence between classical electromagnetism and the Schrödinger equation to the realm of quantum optics, where we showed a straightforward translation of concepts of PT-symmetric evolution with loss and gain not to be possible [1]. However, we could show the first Hong-Ou-Mandel quantum interference of photons in a passive PT-symmetric setting [2]. In order to efficiently model the quantum evolution in these open systems, we are developing analytical tools to solve quantum master equations using group-theoretical methods [3]. First applications include Floquet-PT systems [4] that show a strong reduction of the necessary loss for driving a PT-phase transition.

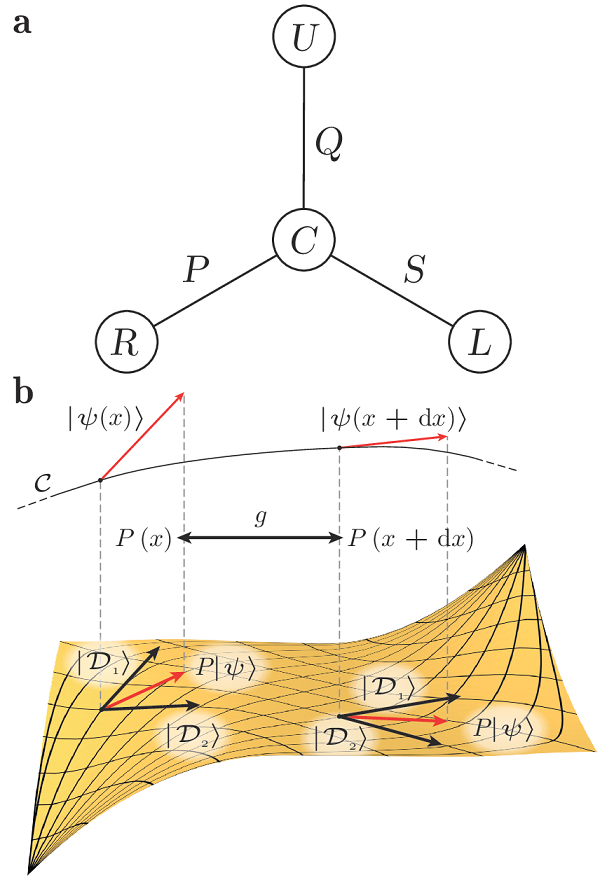
Integrated optical waveguides are highly suitable for implementing adiabatic quantum evolutions by generalizing STIRAP protocols for the evolution in degenerate dark subspaces. We have shown the first non-Abelian geometric quantum gate using an Abelian gauge field [5], and presented an optimal construction using the quantum metric. The concept of holonomic gates can be transferred to non-Hermitian systems [6] which becomes interesting in the framework of non-orthogonal waveguide modes. We also investigate highly degenerate structures for use in holonomic computation [7].